Based on Cage’s description, the steps used to transform each phrase of the melodic line of the first movement of Satie’s Socrate into the equivalent phrase of Cheap imitation were:
- Choose one of the seven “white note” modes
- Choose one of the twelve chromatic transpositions of this mode
- For each note of the Satie (except repeated notes), choose one of the seven scale degrees of the chosen mode to replace the pitch of the original
- If a note repeats in the original phrase, make it also repeat in the new phrase.
To look at this in operation, let’s start at the beginning:

The top staff here is the melodic line of the orchestra music in the Socrate, and the lower staff is the corresponding passage in Cheap imitation. The phrase structure of both works is identical. The rhythms are not quite the same: Cage made the decision to extend notes through the rests. He did this consistently throughout the entire first and second movements. I’ll return to these kinds of decisions later, but I should just note here that this modification of Satie’s rhythms appears to be a personal stylistic choice, not a product of some chance operation that we don’t know about. Cage deliberately composed the first and second movements of Cheap imitation so that they have no silences at all in them, while the third movement introduces gaps as long as two full bars or more.
With the phrases and rhythms essentially the same as Satie’s, that leaves the only changes in the domain of pitch. None of Socrate’s pitches carry over into Cheap imitation, and other than the opening octaves, none of the intervals do, either. The contours of the melody (after the octaves) are subtly different. Just this short example shows another detail about the rules of composition for Cheap imitation that isn’t in Cage’s description: the equivalence of repeated tones and octaves. The Satie opens with a pair of octave A-naturals. Cage treats them as repeated notes, and following his rule #4, he keeps the same pitch (B-natural) through the first four notes. This treatment of octaves as repeated notes works in the other direction as well. According to rule #4, the repeated C-sharps in Socrate in mm. 7–9 should result in repeated E-flats in Cheap imitation. So the jump down an octave in m. 8 and then back up in m. 9 shows that, when preserving repeated notes, Cage allowed octave displacement (at his discretion).
Beyond this detail, how can we validate that Cage’s method worked the way that he described it? With these short phrases of the opening, we can’t reconstruct the choices made: which modes, transpositions, or scale degrees came up randomly. With only one, two, or three scale degrees in a phrase, the possibilities are vast and hence impossible to reverse-engineer. However, longer phrases can provide data to validate the modal method. Looking at the entire first movement, we find, as expected, that no phrase has more than seven diatonic scale degrees in it. This agrees with the modal approach that Cage described.

This example shows a particularly long phrase from the first movement: nine measures with a total of twenty non-repeating notes, each of which would prompt a separate chance operation to select the scale degree. (For reasons I cannot explain, the octave in m. 35 is not preserved) Given that many opportunities, it is not surprising that all seven different scale degrees show up in this example. Specifically the set is A, B-flat, C, D, E, F, and G.
This example points out a problem with Cage’s method, however. The scale used in this phrase is absolutely one of the seven white note modes, but which one? F Ionian? G Dorian? A Phrygian? In fact, it is completely impossible to tell which of the seven modes was selected here. This dilemma arises because of the third step in the process: the replacement of Satie’s pitches with random notes from the chosen mode. The difference between F Ionian and A Phrygian (or any other pair of modes) is a matter of which one is the tonic, the “home base” of the mode as implied by the melodic contours. The notion of a tonic is irrelevant in Cage’s arbitrary melodies, and so the notion of mode is also irrelevant. With no tonic, Cheap imitation is essentially atonal. All that remains is the diatonic scale: the seven-note “white key” scale with no specific starting point, shifting up and down chromatically at each phrase. What this means is that the first step in his process was totally unnecessary. All he really needed to do was select which of the twelve transpositions of the scale to use and then select notes from it.
I wonder whether Cage, once he completed the first movement, realized that his method for composing Cheap imitation was more complex than it needed to be. This could be one explanation for why he changed direction and used a much simpler method for the remaining two movements of the piece. In these movements, the method is down to a single step, repeated every half-measure within every phrase: choose one of the twelve chromatic transpositions to use for this half-measure.
Again, repeated notes are maintained as repeated notes.
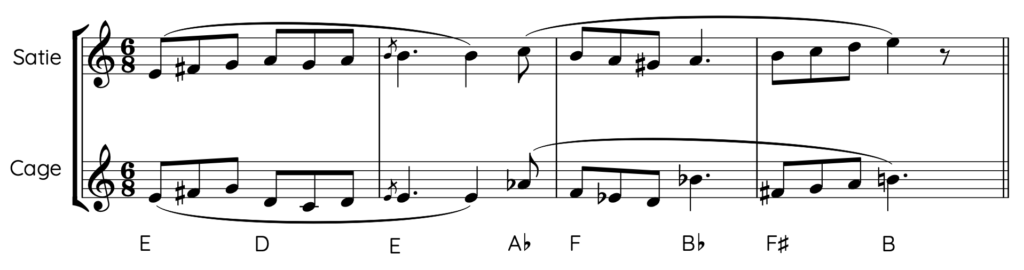
Here are a few measures from the second movement, again showing the original and transformed versions in parallel. The melodic intervals within each half-measure are identical in each version, and I’ve indicated the chance-selected transposition for each half-measure below the Cheap imitation version. In the second measure, note that the rule about repeated notes is maintained here. The three B’s in Socrate become three E’s in Cheap imitation, even though the last of these is in the second half of the measure and otherwise would have prompted the selection of a new transposition. Instead, the new transposition is delayed until the very last note of the bar, when the repetition ends and a new phrase begins.
This rule about preserving repeated notes is changed slightly for the third movement of Cheap imitation. In this movement, repeated notes are only maintained for a half a bar at a time. This means that repeated notes that extend for one or more full measures will change pitch at each half-bar boundary, as seen in this example:

Note here how the repeated notes in measures 50 and 54 that extend to the end of the bar in the Satie shift in pitch in the middle of the bar in the Cage.
So far, so good, but going through the scores of both the second and third movements of Cheap imitation, I encountered a number of pitch anomalies that at first didn’t seem to follow the system’s rules. Here’s an example in the third movement:

In m. 243, by the system’s rules the second note should be a D-sharp, not a B-flat. And in m. 246, the second and third notes should be A and B, not F and G. After studying a number of similar “wrong notes” throughout the third movement, I figured out what was going on. I remembered that Cage’s description of his system states first that he made decisions for every phrase and then, in the second and third movements, for every half-measure. Combining these two rules means that a measure can contain more than two pitch transpositions. In the example above, I have indicated the transpositions selected under the Cheap imitation line. In m. 243, the phrase break on the second beat prompted the system to generate an extra transposition: C-sharp at the beginning of the bar, then B-flat at the start of the new phrase, and finally F at the start of the second half of the bar. A similar situation occurs in m. 246.
The rule of new transpositions at the start of phrases applies in both the second and third movements, although there are relatively few places in the second movement where it comes into play. In the third movement, it can create some more complicated transpositions, as in the example below:
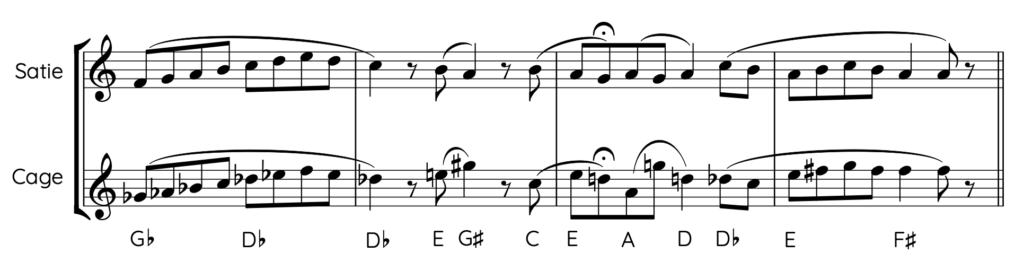
Based on just the half-bar rule, these four measures should include eight transpositions. The new phrase rule, combined with the short phrases found here, increase that total to twelve transpositions (noted in the example).
The method of the second and third movements is simpler than the one used in the first movement and requires far fewer chance operations. It also produces noticeably different music. The first movement is more disjunct: the melody jumps around much more and in unpredictable ways. The second and third movements maintain more of the even, flowing contours of Satie’s writing. They sound more vocal, where the first movement sounds a bit more pianistic. That said, because transpositions are only determined every phrase, the first movement is less chromatic than the later movements, where chromatic shifts mostly happen twice a bar.
The second, simpler process used in Cheap imitation may have been an expedient way to finish the piece under a tight deadline, but it also was the method that Cage continued to use in other “cheap imitations.” Here is an example from the Song Books: the opening of Solo for voice 47 (Cheap imitation No. 4), shown together with its source, the “Queen of the Night” aria from Mozart’s The magic flute.

The method used to transform Mozart’s music is the same one used in the last two movements of Cheap imitation. A new transposition is chosen by chance operations every half-bar, as shown in the example.
To sum up: with Cheap imitation, Cage gave a very general description of the system used to compose it. A close reading of the piece itself—the actual results of that system—confirms the accuracy of the rules Cage listed. At the same time, analysis of the score uncovers considerations and choices that Cage didn’t mention. This gives us a fuller picture of Cheap imitation, but is that all there is to say about the piece? Because Cage’s system only accounts for pitch class and rhythm, it turns out that there is much more to explore. How extensive were Cage’s choices when composing? How did these choices shape the style of the music? And do we have any idea why he made those choices?
This is part of the series Understanding Cheap Imitation
